No Acceleration
Horizontal Acceleration (Friction)
v = 0 m/sec vo = 8.94 m/sec s = ? a = -6.86 m/sec² t = ?
v = vo + at; t = (v - vo)/a = (0 + 8.94 m/sec)/(6.86 m/sec²) = 1.3 sec
v² = vo² + 2as;
s = [v² - vo²]/2a = [0 - (8.94 m/sec)²]/(2)(-6.86 m/sec²) = 5.8 m
v = 0 m/sec vo = ? m/sec s = 30 m a = -3.43 m/sec² t = ?
v² = vo² + 2as;
vo = [v² - 2as]½ = [0 + (2)(3.43 m/sec²)(30 m)]½ = 14.35 m/sec
v = vo + at; t = (v - vo)/a = (0 + 14.35 m/sec)/(3.43 m/sec²) = 4.18 sec
v = 0 m/sec vo = ? m/sec s = ? m a = -4.86 m/sec² t = 2.1 sec
v = vo + at; vo = v - at = 0 + (4.86 m/sec²)(2.1 sec) = 10.2 m/sec
v² = vo² + 2as;
s = [v² - vo²]/2a = [0 - (10.2 m/sec)²]/(2)(-4.86 m/sec²) = 10.7 m
Vertical Acceleration (Gravity)
v = -20.0 m/sec vo = ? m/sec s = ? m a = -9.8 m/sec² t = 2.0 sec
vo = v - at = (-20.0 m/sec) + (9.8 m/sec²)(2.0 sec) = -0.4 m/sec
v² = vo² + 2as;
s = [v² - vo²]/2a = [(-20 m/sec)² - (-0.4 m/sec)²]/(2)(-9.8 m/sec²) = -20.4 m
From start to max:
v = 0 m/sec vo = ? m/sec s = 10 m a = -9.8 m/sec² t = ? sec
v² = vo² + 2as; vo = [v² - 2as]½ = [0 - (2)(-9.8 m/sec²)(10 m)]½ = 14 m/sec
v = vo + at; t = (v - vo)/a = (0 - 14 m/sec)/(-9.8 m/sec²) = 1.43 sec
Total time is the time to go to max plus the time to come back down:
2(1.43 sec) = 2.86 sec
v = ? m/sec vo = 0 m/sec s = ? m a = -9.8 m/sec² t = 4.0 sec
s = vot + ½at² = 0 + ½(-9.8 m/sec²)(4 sec)² = -78.4 m
The well is 78.4 m deep.
Also:
v = vo + at = (0 m/sec) - (9.8 m/sec²)(4.0 sec) = -39.2 m/sec
1st Time: v = ? m/sec vo = 0 m/sec s = ? m a = -9.8 m/sec² t = 3.0 sec
s = vot + ½at² = 0 + ½(-9.8 m/sec²)(3 sec)² = -44.1 m
2nd Time: v = ? m/sec vo = -3.1 m/sec s = ? m a = -9.8 m/sec² t = 2.7 sec
s = vot + ½at² = (-3.1 m/sec)(2.7 sec) + ½(-9.8 m/sec²)(2.7 sec)² = -44.1 m
Both calculations say the building is 44.1 m high. The model works!
v = -50 m/sec vo = 0 m/sec s = ? m a = -9.8 m/sec² t = ? sec
t = (v - vo)/a = [(-50 m/sec) - 0]/(-9.8 m/sec²) = 5.1 sec
s = vot + ½at² = 0 + ½(-9.8 m/sec²)(5.1 sec)² = -127.5 m
Horizontal and Vertical Acceleration (Projectiles)
|
Here are some mathematical relationships that will be helpful for some of these two dimensional problems. | 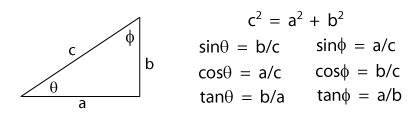
|
Calculate components of the initial velocity in x and y.
In y: voy = vosin25° = (3 m/sec)(sin25°) = 1.27 m/sec
In x: vox = vocos25° = (3 m/sec)(cos25°) = 2.72 m/sec
| y | x | |
| v | ? m/sec | 2.72 m/sec |
| vo | 1.27 m/sec | 2.72 m/sec |
| s | -0.5 m | ? m |
| a | -9.8 m/sec | 0 m/sec |
| t | ? sec | ? sec |
Make a table from jump to water.
Solve for v in the y-direction, then solve for t in the y-direction. Use the time from the y-direction in the x-direction to determine the distance in the x-direction.
vy = [voy² + 2aysy]½ = [(1.27 m/sec)² + (2)(-9.8 m/sec²)(-0.5 m)]½
= -3.38 m/sec
t = (vy - voy)/ay = [(-3.38 m/sec) - 1.27 m/sec]/(-9.8 m/sec²) = 0.474 sec
sx = voxt + ½at² = (2.72 m/sec)(0.474 sec) + 0 = 1.29 m
| y | x | |
| v | ? m/sec | ? m/sec |
| vo | 0 m/sec | ? m/sec |
| s | -1000 m | 2000 m |
| a | -9.8 m/sec | 0 m/sec |
| t | ? sec | ? sec |
Make a table from drop to ground.
Solve for v in the y-direction, then solve for t in the y-direction. Use the time from the y-direction in the x-direction to determine the velocity in the x-direction.
vy = [voy² + 2aysy]½ = [0 + (2)(-9.8 m/sec²)(-1000 m)]½
= -140 m/sec
t = (vy - voy)/ay = [(-140 m/sec) - 0]/(-9.8 m/sec²) = 14.3 sec
vox = sx/t = (2000 m)/(14.3 sec) = 140 m/sec
For the final velocity:
v = [(vx)² + (vy)²]½ = [(-140 m/sec)² + (140 m/sec)²]½ = 198 m/sec
θ = tan-1[(vx)/vy)] = tan-1(140/140) = 45°
v = 198 m/sec at an angle of 45° below horizontal.
Get the initial velocity from the initial components.
v0 = [(vox)² + (voy)²]½ = [(160 m/sec)² + (75 m/sec)²]½ = 176.7 m/sec
θ = tan-1[(voy)/vox)] = tan-1(75/160) = 25.1°
The initial velocity is 176.7 m/sec at 25.1° above horizontal.
| y | x | |
| v | 0 m/sec | 160 m/sec |
| vo | 75 m/sec | 160 m/sec |
| s | ? m | ? m |
| a | -9.8 m/sec | 0 m/sec |
| t | ? sec | ? sec |
Make a table going from start to max.
Solve for s in the y-direction to get the maximum height.
sy = [(vy)² - (voy)²)]/[2ay] = [(0 - (75 m/sec)²)]/[2(-9.8 m/sec²)] = 287 m
t = (vy - voy)/ay = [(0 m/sec) - 75 m/sec]/(-9.8 m/sec²) = 7.65 sec (to max)
The total time is 2(7.65 sec) = 15.3 sec
For the distance in x (the range): sx = vot = (160 m/sec)(15.3 sec) = 2449 m
| y | x | |
| v | vsinθ m/sec | vcosθ m/sec |
| vo | vosinθ m/sec | vocosθ m/sec |
| s | 0 m | 30 m |
| a | -9.8 m/sec | 0 m/sec |
| t | t sec | t sec |
Make a table going from start to end.
This will require solving two equations and two unknowns. Solve for t in both the x-direction and the y-direction, then set those expressions equal to each other.
In x: 30 m = (vosinθ m/sec)(t) or t = (30 m)/(vosinθ m/sec)
In y: sy = voyt + ½ayt² = [voy + ½ayt]t
0 = [voy + ½ayt]t says that either t = 0 or [voy + ½ayt] = 0
Time equal to zero is before it starts, so the second expression is needed to agree with the physical situation. So, from y:
t = -voy/½a = -(vosinθ)/½a
That means:
-(vosinθ)/½a = (30 m)/(vosinθ m/sec) or -(vo²)(sinθ)(cosθ) = ½a(30)
vo = -[½a(30)]/[(sinθ)(cosθ)]½ = [(15 m)(9.8 m/sec²)]/[(sin40°)(cos40°)]½
= 17.3 m/sec
From part (a):
vox = vocosθ m/sec = (17.3 m/sec)cos40° = 13.3 m/sec
voy = vosinθ = (17.3 m/sec)sin40° = 11.12 m/sec
Make a table going from start to max.
| y | x | |
| v | 0 m/sec | vx = vox m/sec |
| vo | 11.12 m/sec | 13.3 m/sec |
| s | ? m | 15 m |
| a | -9.8 m/sec | 0 m/sec |
| t | t sec | t sec |
sy = [(vy)² - (voy)²)]/[2ay] = [(0 - (11.2 m/sec)²)]/[2(-9.8 m/sec²)]
= 6.4 m
t = (vy - voy)/ay = [(0 m/sec) - 11.2 m/sec]/(-9.8 m/sec²) = 1.14 sec (to max)
The total time is 2(1.14 sec) = 2.28 sec
Make a table going from start to end.
| y | x | |
| v | vsinθ m/sec | vx = vox m/sec |
| vo | vosinθ m/sec | vocosθ m/sec |
| s | -15 m | 10 m |
| a | -9.8 m/sec | 0 m/sec |
| t | ? sec | ? sec |
Solve for the time in x and substitute the resulting expression into the distance expression for y.
In x: t = (10 m)/(vocos40°)
sy = (voy)t + ½at²
Substiute t from above and then solve for the initial velocity, vo.
-15 m = (vo)(sin40°)[(10 m)/(vocos40°)] + ½(-9.8 m/sec²)[(10 m)/(vocos40°)]²
vo = 5.975 m/sec; voy = 3.84 m/sec; vox = 4.58 m/sec;
t = (10 m)/(4.58 m/sec) = 2.18 sec
vy = 3.84 m/sec - (9.8 m/sec²)(2.18 sec) = -17.52 m/sec
v = [(4.58 m/sec)² + (-17.52 m/sec)²]½ = 18.1 m/sec
θ = tan-1(-17.52/4.58) = 75.3°
t = 2.18 sec, v = -17.52 m/sec at 75.3° below the positive x-axis.
| y | x | |
| v | 0 m/sec | vx = vox m/sec |
| vo | ? m/sec | ? m/sec |
| s | ? m | ? m |
| a | -9.8 m/sec | 0 m/sec |
| t | 1.05 sec | 1.05 sec |
Symmetry makes this problem much easier.
Make a table going from start to max.
0 = voy + at; voy = -at = (9.8 m/sec²)(1.05 sec) = 10.29 m/sec
vo = (voy)/sinθ = (10.29 m/sec)/sin10° = 59.3 m/sec at 10°
vox = (vo)(cosθ) = (59.3 m/sec)(cos10°) = 58.4 m/sec
Because of the symmetry of this problem the final velocity in y will be the negative of the initial velocity in y. The velocity in x remains constant. θ = tan-1(-10.29/58.4) = -10°
v = [(vx)² + (vy)²]½ = 59.3 m/sec at -10°
sx = (58.4 m/sec)(2.1 sec) = 123 m
EXTRA PROBLEMS
- Answer
"Your students might immediately want to plug the launch speed and launch angle into the range equation,R is the range, or the distance in x. My questions is: can you derive this equation using our method of doing these problems?R=(v02 sin2θ)/g,
where v0 is the launch speed, g is the acceleration due to gravity, and θ is the vertical launch angle."
- Answer

Static and Dynamic Equilibrium Homework
- A 3.6 kg flower pot hangs from a tree suspended by a rope. What is the tension in the rope?
Answer
Force up = Force down
T = mg = (3.6 kg)(9.8 m/sec²) = 35.28 N
-
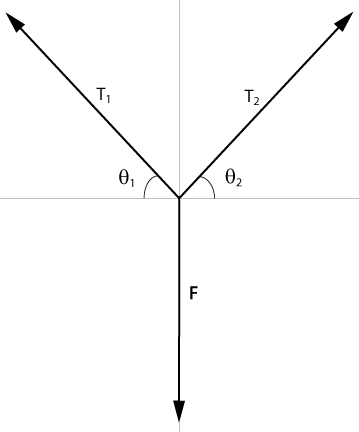
If θ1 = θ2 = 25° and F = 5.6 N, what are the tensions T1 and T2?
Answer
T1x = T2x and T1y + T2y = F
T1cosθ1 = T2cosθ2 and T1sinθ1 + T2sinθ2 = F
T2 = (T1cosθ1)/cosθ2 and T1sinθ1 + [(T1cosθ1)/cosθ2]sinθ2 = F
T1[sinθ1 + [(cosθ1)(tanθ2)] = F ⇒ T1 = F/[sinθ1 + [(cosθ1)(tanθ2)]
T1 = (5.6 N)/[sin25° + [(cos25°)(tan25°)] = 6.63 N
T2 = (T1cosθ1)/cosθ2 = (6.63 N)(cos25°)/(cos25°) = 6.63 N
-
If the tensions T1 and T2 are both equal to 38 N and θ1 = θ2 = 15°, what is the force F?
Answer
T1x = T2x and T1y + T2y = F
F = T1y + T2y = T1sinθ1 + T2sinθ2
F = (38 N)(sin15°) + (38 N)(sin15°) = 19.67 N
-
If F = 12 N and the tensions T1 and T2 are both equal to 22 N, what are the angles θ1 and θ2?
Answer
T1x = T2x and T1y + T2y = F
⇒ T1cosθ1 = T2cosθ2 and T1sinθ1 + T2sinθ2 = F
T1cosθ1 = T2cosθ2 and T1 = T2 ⇒ cosθ1 = cosθ2 ⇒ θ1 = θ2
Tsinθ1 + Tsinθ1 = F ⇒ sinθ1 = F/(2T)
θ1 = sin-1[F/2T] = sin-1[(12 N)/{2(22 N)}] = 15.8° = θ2
-
If θ1 = 20°, θ2 = 30°, T1 = 8 N, and T2 = 5 N, what is the force F?
Answer
F = T1y + T2y = T1sinθ1 + T2sinθ2
F = (8 N)(sin20°) + (5 N)(sin30°) = 5.24 N
-
If F = 40 N, θ2 = 56.31°, and T2 = 30 N, what are T1 and θ1?
Answer
T1x = T2x and F = T1y + T2y
⇒ T1cosθ1 = T2cosθ2 and F = T1sinθ1 + T2sinθ2
⇒ T1 = T2cosθ2/cosθ1 and F = (T2cosθ2/cosθ1)sinθ1 + T2sinθ2
⇒ F = T2cosθ2tanθ1 + T2sinθ2 ⇒ tanθ1 = (F - T2sinθ2)/T2cosθ2
⇒ tanθ1 = [(40 N) - (30 N)sin56.31°]/[(30 N)cos56.31°] = 0.9037
θ1 = tan-10.9037 = 42.1°
T1 = [F - T2sinθ2]/sinθ1 = [(40 N) - (30 N)sin56.31°]/sin42.1° = 22.43 N
-
A 600 N gymnast, in a display of upper body and hand strength, brought both rings together and held them in one hand. At that point there were 30° between the ropes (assume them to be the same length). What tension is in each rope?
Answer
T1x = T2x and F = T1y + T2y
⇒ T1cosθ1 = T2cosθ2 and F = T1sinθ1 + T2sinθ2
T1 = T2 = T and θ1 = θ2 = θ = 90° - 15° = 75°
⇒ F = Tsinθ + Tsinθ = 2Tsinθ
⇒ T = F/(2sinθ) = (600 N)/(2sin75°) = 311 N
-
One at a time, members of a scout troop cross a river by moving hand over hand on a horizontal rope attached to trees on opposite sides of the river that are 16 m apart. What will the tension in the rope be on each side of a 500 N scout at a horizontal distance of 4 m if the rope is pulled down 1 m? (Answer: 1505 N and 1546 N)
Answer
Using the distances:
tanθ1 = (1 m)/(4 m) ⇒ θ1 = 14° and tanθ2 = (1 m)/(12 m) ⇒ θ2 = 4.76°
T1x = T2x and F = T1y + T2y
⇒ T1cosθ1 = T2cosθ2 and F = T1sinθ1 + T2sinθ2
⇒ T1 = T2cosθ2/cosθ1 and F = (T2cosθ2/cosθ1)sinθ1 + T2sinθ2
⇒ F = T2cosθ2tanθ1 + T2sinθ2 ⇒ T2 = F/[cosθ2tanθ1 + sinθ2]
⇒ T2 = (500 N)/[cos4.76°tan14° + sin4.76°] = 1509 N
⇒ T1 = T2cosθ2/cosθ1 = T1 = (1509 N)cos4.76°/cos14° = 1549 N
-
A 35 N block slides down a 25° ramp at a constant speed of 1.0 m/sec. How much force is keeping the block from accelerating?
Answer
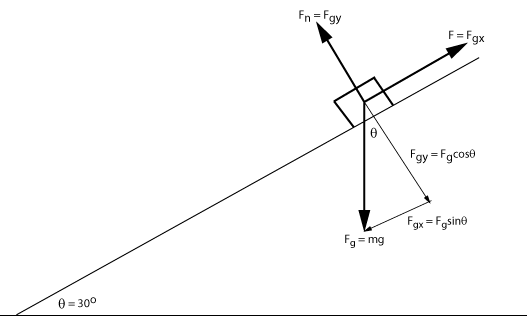
F = (mg)sinθ = (35 N)sin25° = 14.8 N
Refer to the diagram below for the next few problems.
Net Force Problems
-
A 150 g mass and a 400 g mass are hanging from the ends of a string that is over a friction-free pulley. What is the acceleration of the masses and what is the tension in the string?
Answer
Whole System:
netForce = Fg2 - Fg1 = ma
= (0.4 kg)(9.8 m/sec²) - (0.15 kg)(9.8 m/sec²) = 2.45 N
= 2.54 N = ma
a = (2.45 N)/(0.55 kg) = 4.45 m/sec²
Isolate the 0.4 kg Mass:
netForce = Fg2 - T2 = ma
T2 = Fg2 - ma = (0.4 kg)(9.8 m/sec²) - (0.4 kg)(4.45 m/sec²)
T2 = 2.14 N
-
A 3 kg box is placed on a friction-free horizontal table and connected by a string over a friction-free pulley to a 2 kg box that is hanging off of the edge of the table. What is the acceleration of this system and what is the tension in the string?
Answer
Whole System:
netForce = Fg2 = (m1 + m2)a
= (2 kg)(9.8 m/sec²) = 19.6 N = (5 kg)a
a = (19.6 N)/(5 kg) = 3.92 m/sec²
Isolate the 2 kg Mass:
netForce = Fg2 - T = m2a
T = Fg2 - m2a = (2 kg)(9.8 m/sec²) - (2 kg)(3.92 m/sec²)
T = 11.76 N
-
A 20 N block accelerates at 2.34 m/sec² down a 30° ramp. How much friction is slowing down the block? What is the coefficient of friction in this case?
Answer
Fgx = Fgsinθ ; Fgy = Fgcosθ ; Fn = Fgy ; fk = μkFn
m = (20 N)/(9.8 m/sec²) = 2.04 kg
x-axis (along the incline):
netForce = Fgx - fk = ma
fk = Fgx - ma = (20 N)sin30° - (2.04 kg)(2.34 m/sec²) = 5.22 N
y-axis (perpendicular to the plane):
fk = μkFn
μk = fk/Fn = (5.22 N)/[(20 N)cos30°]
μk = 0.3
-
A 5 kg mass is on a 25° ramp and has a coefficient of kinetic friction of 0.11 with the ramp. A string is pulling on this 5 kg mass due to a second mass that is connected to the other end of the string and hanging over the end of the ramp (using a friction-free pulley). If the second, hanging mass is 12 kg, what is the acceleration and what is the tension in the string?
Answer
F12 = (12 kg)(9.8 m/sec²) = 117.6 N ; F5 = (5 kg)(9.8 m/sec²) = 49 N
F5x = F5sinθ = (49 N)sin25° = 20.71 N ; F5y = F5cosθ = (49 N)cos25° = 44.41 N
Fn = F5y = 44.41 N ; fk = μkFn = (0.11)(44.41 N) = 4.88 N
Whole System:
netForce = F12 - fk - F5x = (m12 + m5)a
= 117.6 N - 4.88 N - 20.71 N = 92 N = (17 kg)a
a = (92 N)/(17 kg) = 5.41 m/sec²
Isolate the 12 kg Mass:
netForce = F12 - T = m12a
T = F12 - m12a = 117.6 N - (12 kg)(5.41 m/sec²) = 52.68 N
Centripetal Force Problems
-
A car of mass 1.5 x 10³ kg rounds a circular turn of radius 20 m. If the road is flat and the coefficient of friction is 0.50 between the tires and the road, how fast can the car travel without skidding?
Answer
fs = Fc ; fs = μsFn ; Fc = mv²/r ; Fn = mg
⇒ μsmg = mv²/r ⇒ μsg = v²/r
⇒ v = [rμsg]½ = [(0.5)(9.8 m/sec²)(20 m)]½ = 9.9 m/sec
-
A race track designed for average speeds of 240 km/h is to have a turn with a radius of 975 m. To what angle must the track be banked so that cars traveling 240 km/h have no tendency to slip sideways?
Answer
See example #2 in the Centripetal Force section of Newton's Laws for a diagram.
Fgx = Fcx
Fgsinθ = Fccosθ
⇒ tanθ = Fc/Fg = (m)(v²/r)/mg = v²/rg
= [(240 km/h)(1000 m/km)(1 h/3600 sec)]² /(9.8 m/sec²)(975 m)
= .466
⇒ θ = tan-1(.466) = 24.9°
-
What is the maximum speed of a 1500 kg car at the top of a hill that has a radius of curvature of 30 m?
Answer
At the top of the hill the gravitational force pulling the car down must at least equal the centripetal force that would make the car go straight (at a tangent to the curve, away from the center). The maximum speed would be when those two forces are equal: Fg = Fc.
⇒ mg = mv²/r
⇒ v =[rg]½ = [(30 m)(9.8 m/sec²)]½ = 17.15 m/sec²
EXTRA PROBLEMS
- A 20 N box sits on a 40 degree inclined plane and is connected to a rope that goes over a pulley at the top end of the inclined plane and is then connected to a 2.9 kg weight that hangs freely. (a) How fast will the system accelerate and (b) what will the tension in the rope be?
- Two masses, M1 = 2 kg and M2 = 3 kg, are suspended on opposite ends of a rope that has been placed over a pulley. What is the acceleration and what is the tension in the rope?
- Calculate the acceleration when a 20 N sled is being pulled over smooth ice with a horizontal force of 3 N.
- Find the tension in the rope and the acceleration when a 6 g mass and an 8 g mass are suspended from opposite ends of a rope that is placed over a pulley.
- A 60 N box is placed on a 35 degree inclined plane and connected to a rope that provides a constant upward force of 10 N. What is the acceleration of the box if the coefficient of kinetic friction is 0.3?
-
A 5 kg mass is accelerated up a 35 degree inclined plane by the tension in a rope that goes over a pulley and is connected to a second 3 kg mass that hangs freely. If the system accelerates at 0.14 m/sec², what is the coefficient of kinetic friction between the 5 kg mass and the inclined plane? What is the tension in the rope?
- Answer

-
If F = 40 N, T1 = 20 N, and T2 = 30 N, what are the angles θ1 and θ2?
- Answer

-
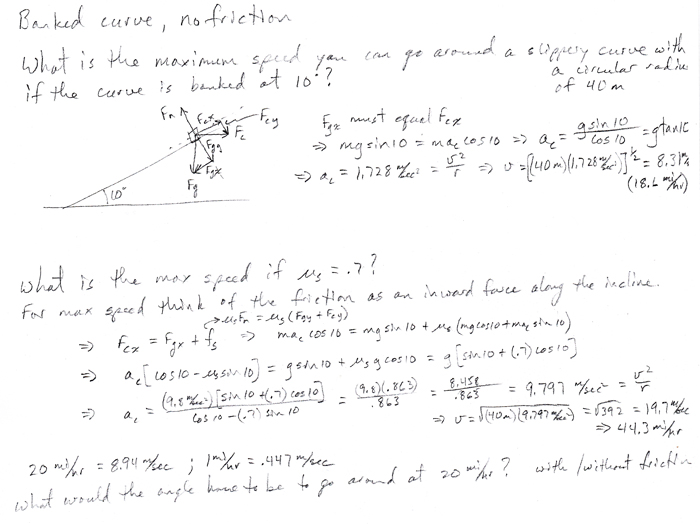
What is the maximum speed you can go around a slippery curve with a circular radius of 40 m if the curve is banked at 10°?
- Answer
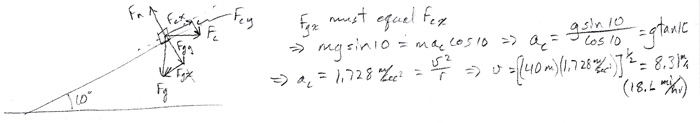
-
What is the maximum speed you can go around a curve with a circular radius of 40 m if the curve is banked at 10° and there is friction with μs = 0.7?
- Answer
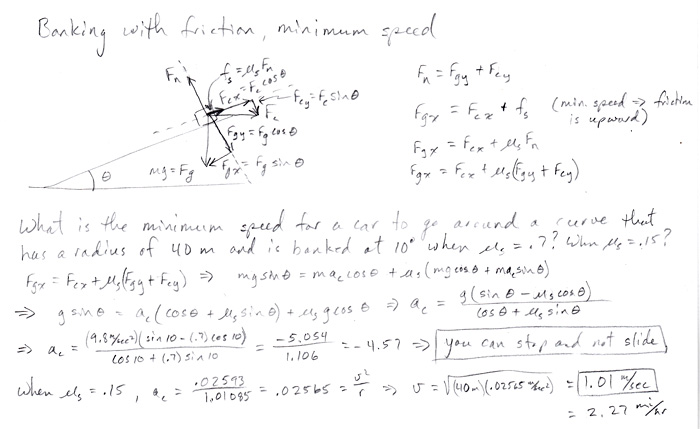
-
What is the minimum speed you can go around a curve with a circular radius of 40 m if the curve is banked at 10° and there is friction with μs = 0.7? What if μs is only 0.15?
- Answer
Work and Conservation Laws
-
How much work is done when a 0.75 kg book is raised 1.5m?
Answer
W = Fs = mgh = (0.75 kg)(9.8 m/sec²)(1.5 m) = 11.03 J
-
How much work is done when a 1.5 kg box slides down a 20° friction-free incline a distance of 2 m?
Answer
W = Fs = Fxsx = [(1.5 kg)(9.8 m/sec²)(sin20°)](2 m) = 10.06 J
-
What distance was traveled if a 25 N force required 18 J of work to move the object?
Answer
W = Fs ⇒ s = W/F = (18 J)/(25 N) = 0.72 m
-
What is the final velocity when sliding down a 20° incline if: vi = 7 m/sec, s = 2 m, and W = 0?
Answer
KEi + PEi = KEf + PEf + W ⇒ ½mvi² + mghi = ½mvf² + mghf + Fs
Set h = 0 at the bottom of the incline.
⇒ ½m(7 m/sec)² + m(9.8 m/sec²)[(2 m)(sin20°)] = ½mvf² + 0 + 0
The mass cancels out
⇒ vf² = 2{½(7 m/sec)² + (9.8 m/sec²)[(2 m)(sin20°)]}
⇒ vf = (62.41 m²/sec²)½ = 7.9 m/sec
-
A ball starting from rest rolls down a friction-free "roller-coaster" track starting at a height of 12 m and goes through a dip at a height of 3 m and then goes back up on top of a "hill" at a height of 8 m. What is the speed at the top of the 8 m hill?
Answer
KEi + PEi = KEf + PEf + W ⇒ ½mvi² + mghi = ½mvf² + mghf + Fs
Set h = 0 at 8 m. Friction-free ⇒ W = 0. From rest ⇒ vi = 0.
⇒ 0 + m(9.8 m/sec²)(4 m) = ½mvf² + 0 + 0
The mass cancels out
⇒ vf = [2{9.8 m/sec²)(4 m)]½ = 8.85 m/sec
-
What is the final velocity when a 3.3 kg mass slides down a 50° incline if: vi = 0, s = 3.3 m, µk = 0.12?
Answer
KEi + PEi = KEf + PEf + W ⇒ ½mvi² + mghi = ½mvf² + mghf + W
Set h = 0 at the bottom of the incline. Fg = mg
⇒ W = fk(s) = µkFn(s) = µkFgy(s) = µk(mgcosθ)(s)
With this W the mass will still cancel out. hi = (3.3 m)(sin50°) = 2.528 m.
⇒ 0 + (9.8 m/sec²)(2.528 m) = ½vf² + 0 + (0.12)(9.8 m/sec²)(cos50°)(3.3 m)
⇒ 0 + 24.774 m²/sec² = ½vf² + 0 + 2.495 m²/sec²
⇒ vf = [2(24.774 m²/sec² - 2.495 m²/sec²)]½ = 6.68 m/sec
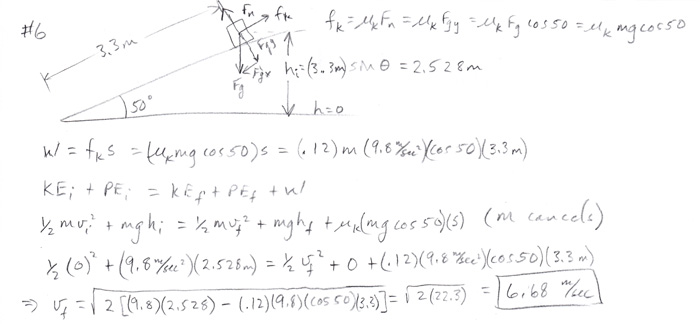
-
A slide for young children is 1.75 m high at an angle of 40° from the horizontal with an additional horizontal length of slide at a height of 0.25 m from the ground. (a) If the slide is friction-free, what is the velocity of the child at the bottom of the incline, starting from rest? (b) If the coefficient of friction between the child and the horizontal part of the slide is 0.11, how long should the horizontal section be to stop the child completely?
Answers: Part (a):
KEi + PEi = KEf + PEf + W ⇒ ½mvi² + mghi = ½mvf² + mghf + Fs
Set h = 0 at the bottom of the incline. hi = 1.75 m - 0.25 m = 1.5 m. W = 0.
Fg = mg Fgx = mgsinθ
With W = 0 the mass will cancel out.
⇒ 0 + (9.8 m/sec²)(1.5 m) = ½vf² + 0 + 0
⇒ vf = [2(9.8 m/sec²)(1.5 m)]½ = 5.42 m/sec
Part (b): Start: After the incline, End: where it stops on the horizontal section.KEi + PEi = KEf + PEf + W ⇒ ½mvi² + mghi = ½mvf² + mghf + W
Set h = 0 at the bottom of the incline. hi = hf = 0. vi = 5.42 m/sec. vf = 0.
W = Fs = fks = Fnµks = Fgµks = (mg)(µk)(s)
⇒ ½mvi² + 0 = 0 + 0 + (mg)(µk)(s)
Divide both sides by the mass and multiply both sides by 2.
⇒ vi² + 0 = 0 + 0 + (2)(g)(µk)(s)
⇒ s = (vi²)/[(2)(g)(µk)] = (5.42 m/sec)²/[(2)(9.8 m/sec²)(0.11)] = 13.6 m
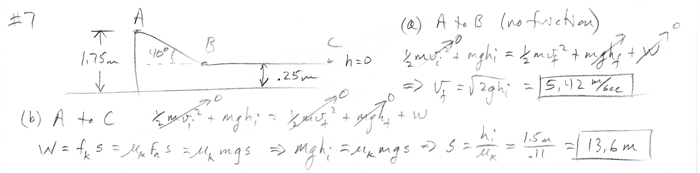
-
What will be the final velocity of a rock that is thrown off of a 15 m cliff with a speed of 20 m/sec at an angle of 30° above the horizontal? Use conservation of energy.
Answer
KEi + PEi = KEf + PEf + W ⇒ ½mvi² + mghi = ½mvf² + mghf + Fs
Set h = 0 at the bottom. Friction-free (ignore air friction) ⇒ W = 0.
vix = (20 m/sec)(cos30°) = 17.32 m/sec viy = (20 m/sec)(sin30°) = 10 m/sec
The mass again cancels out. In the vertical direction:
⇒ ½(10 m/sec)² + (9.8 m/sec²)(15 m) = ½vfy² + 0 + 0
⇒ vfy = {2[(50 m²/sec²) + (147 m²/sec²)]}½ = 19.8 m/sec
vf = [vfx² + vfy²]½ = [(17.32 m/sec)² + (19.8 m/sec)²]½ = 26.3 m/sec
-
A car runs out of gas just as it goes over the top of a hill. If the car is traveling at 75 km/hr at the top of the hill and the hill is 20 m high: (a) Will the car make it to the top of the next hill, which is 25 m high? (b) What is the minimum speed that the car can have and still make it to the top of the next hill?
Answer
KEi + PEi = KEf + PEf + W ⇒ ½mvi² + mghi = ½mvf² + mghf + Fs
Set h = 0 at 20 m. hf = 5 m. Assume friction-free ⇒ W = 0.
vi = (75 km/hr)(1000 m/1 km)(1 hr/3600 sec) = 20.83 m/sec
The mass again cancels out. The velocity at the top of the second hill must be at least zero (a). The minimum speed is when the final velocity is zero (b).
⇒ ½(20.83 m/sec)² = ½vf² + (9.8 m/sec²)(5 m) + 0
⇒ vf = [2{(217 m²/sec²) - (49 m²/sec²)}]½ = 18.33 m/sec
⇒ The car will go over the top of the hill at 18.33 m/sec. (Part a)
Part b: vf = 0 ⇒ vi = [2ghf]½ = 9.9 m/sec
-
A 500 N boy slides down a 30° incline starting from rest. His final velocity is 1.0 m/sec after going 5.0 m down the incline. What is the coefficient of friction between the boy and the incline?
Answer
KEi + PEi = KEf + PEf + W ⇒ ½mvi² + mghi = ½mvf² + mghf + Fs
Set h = 0 at 5 m down the ramp. hi = (5 m)sin30° = 2.5 m. hf = 0 m.
vi = 0. vf = 1 m/sec. m = Fg/g = (500 N)/(9.8 m/sec²) = 51 kg
W = Fs = (Fgx - fk)s = (Fgsinθ - Fnµk)s = (Fgsinθ - Fgyµk)s
= (Fgsinθ - [Fgcosθ]µk)s = Fg[sinθ]s - Fg[cosθ]µks
= (500 N)(sin30°)(5 m) - (500 N)(cos30°)(µk)(5 m) = 1250 J - (2165 J)µk
⇒ 0 + mghi = ½mvf² + 0 + W
⇒ 0 + (51 kg)(9.8 m/sec²)(2.5 m) = ½(51 kg)(1 m/sec)² + 0 + 1250 J - (2165 J)µk
⇒ µk = [½(51 kg)(1 m/sec)² + 1250 J - (51 kg)(9.8 m/sec²)(2.5 m)]/(2165 J) = 0.012
-
A 400 g air track car and a 350 g air track car are fitted with velcro so that they remain together upon collision. If the 400 g car is traveling at 0.12 m/sec and collides with the 350 g car when it is at rest, what will be the final velocity of the two cars (which remain together)?
Answer
pi = pf ⇒ p1i + p2i = p1f + p2f ⇒ m1v1i + m2v2i = m1v1f + m2v2f
In this case: m1v1i + m2v2i = (m1 + m2)vf
⇒ vf = (m1v1i + m2v2i)/(m1 + m2) = [(0.4 kg)(0.12 m/sec) + 0]/(0.4 kg + 0.35 kg)
⇒ vf = 0.064 m/sec
-
A 400 g air track car and a 350 g air track car are fitted with velcro so that they remain together upon collision. If the 400 g car is traveling at 0.12 m/sec and collides with the 350 g car when it is traveling toward the 400 g car at 0.06 m/sec, what will be the final velocity of the two cars (which remain together)?
Answer
pi = pf ⇒ p1i + p2i = p1f + p2f ⇒ m1v1i + m2v2i = m1v1f + m2v2f
In this case: m1v1i + m2v2i = (m1 + m2)vf
⇒ vf = (m1v1i + m2v2i)/(m1 + m2)
⇒ vf = [(0.4 kg)(0.12 m/sec) + (0.35 kg)(-0.06 m/sec)]/(0.4 kg + 0.35 kg)
⇒ vf = 0.036 m/sec
-
A 55 kg boy and a 45 kg girl face each other on ice skates. The girl pushes the boy, who moves away at a speed of 2 m/sec. What is the girl's speed?
Answer
pi = pf ⇒ p1i + p2i = p1f + p2f ⇒ m1v1i + m2v2i = m1v1f + m2v2f
In this case: 0 = m1v1f + m2v2f
⇒ v2f = -m1v1f/m2
⇒ v2f = -[(55 kg)(2 m/sec)]/(45 kg) = -2.44 m/sec
⇒ v2f = 2.44 m/sec away from the boy
-
A 50 kg girl throws a 1.5 kg physics book horizontally with a speed of 1.0 m/sec while on friction-free roller skates. What is the girl's velocity (speed and direction)?
Answer
pi = pf ⇒ p1i + p2i = p1f + p2f ⇒ m1v1i + m2v2i = m1v1f + m2v2f
In this case: 0 = m1v1f + m2v2f
⇒ v2f = -m1v1f/m2
⇒ v2f = -[(1.5 kg)(1 m/sec)]/(50 kg) = -0.03 m/sec
⇒ v2f = 0.03 m/sec away from the book
-
Two giants are playing modified ice hockey (no friction). One giant hits a 45 kg puck so it is moving at 2 m/sec along a positive x-axis. A second giant, who is over and down a ways, smacks a 60 kg puck directly up the y-axis at 3 m/sec. The two pucks collide, stick together and remain together as they move. Determine the final velocity (magnitude and direction) of the combined pucks.
Answer
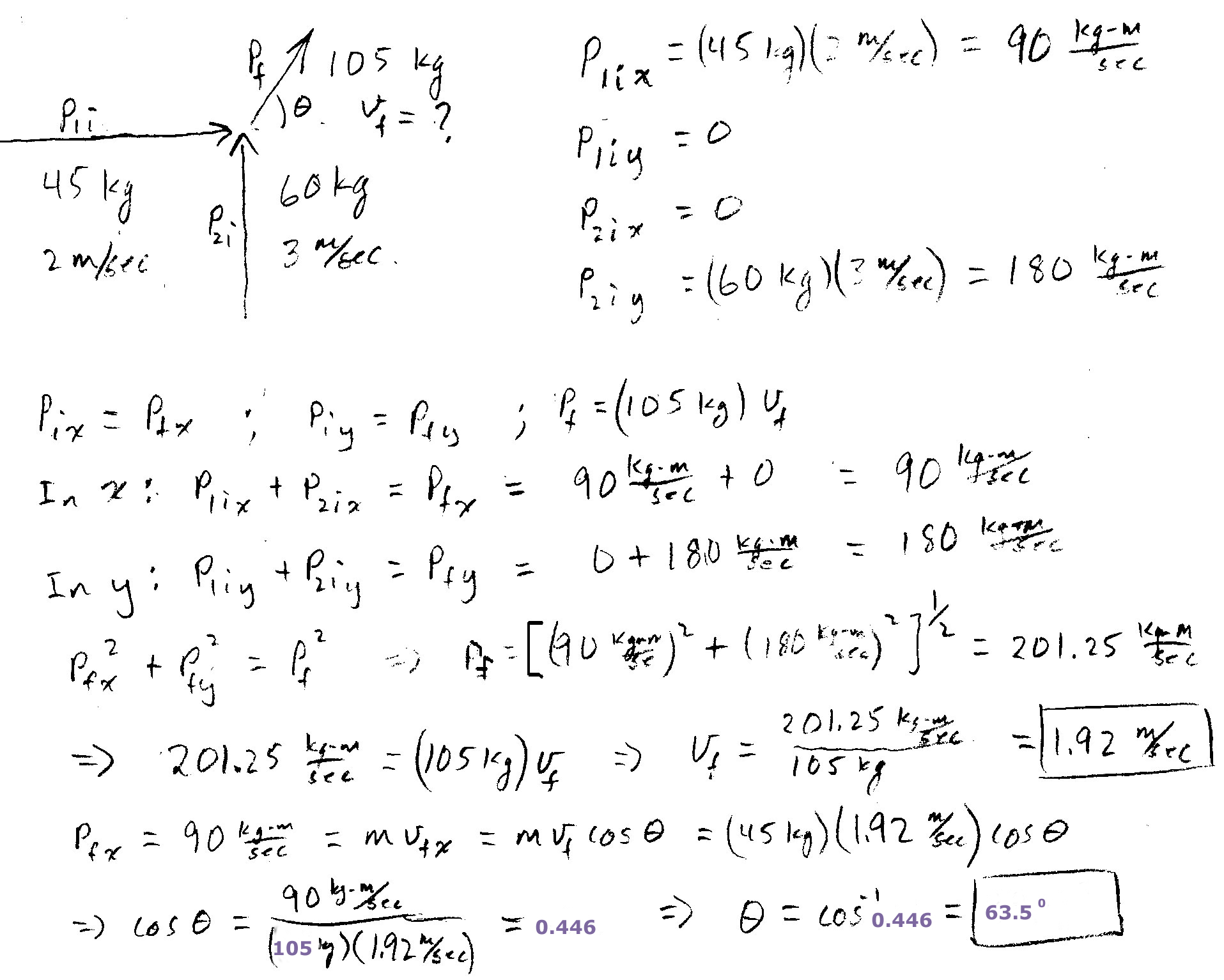
Conservation of Energy and Momentum Problems
- Two billiard balls are traveling toward each other. A 500g billiard ball going 0.15 m/sec collides head-on and elastically with a 350 g billiard ball going 0.2 m/sec. What are the final velocities of each ball?
AnswerConservation of Energy ⇒ KEi + PEi = KEf + PEf + W
Set h = 0 at the height of the billiard balls. ⇒ All PE = 0. W = 0 (elastic).
⇒ ½m1v1i² + ½m2v2i² = ½m1v1f² + ½m2v2f² ⇒ m1v1i² + m2v2i² = m1v1f² + m2v2f²
⇒ (0.5 kg)(0.15 m/sec)² + (0.35 kg)(-0.2 m/sec)² = 0.02525 J = m1v1f² + m2v2f²
⇒ 0.02525 = (0.5)v1f² + (0.35)v2f² (From Cons. of Energy, two unknowns)
Conservation of Momentum ⇒ m1v1i + m2v2i = m1v1f + m2v2f
⇒ (0.5 kg)(0.15 m/sec) - (0.35 kg)(0.2 m/sec) = 0.005 kg-m/sec = m1v1f + m2v2f
⇒ 0.005 = (0.5)v1f + (0.35)v2f (From Cons. of Momentum, two unknowns)
Substitute v2f = (0.005 - (0.5)v1f)/(0.35) into Cons. of Energy Eqn.
⇒ 0.02525 = (0.5)v1f² + (0.35)[(0.005 - (0.5)v1f)/(0.35)]²
⇒ (1.2143)v1f² - (0.01429)v1f - 0.02518 = 0 ⇒ v1f = +0.15 m/sec or -0.138 m/sec
⇒ v1f = -0.138 m/sec and v2f = 0.211 m/sec
- A 10 g bullet is shot horizontally at a 1.5 kg wooden block suspended from the ceiling. The bullet is embedded into the wooden block. The bullet and block then swing in an arc to a height of 12 cm. What is the velocity of the bullet before hitting the wooden block? How much energy was given off when the bullet was embedded into the wooden block?
AnswerSee webpage for more details. Set h = 0 at bottom.
After bullet is in the block to maximum height.
⇒ ½(m1 + m2)vi² + 0 = 0 + (m1 + m2)ghf + 0
⇒ vi = [(2)([m1 + m2]ghf)/(m1 + m2)]½ = [(2)(9.8 m/sec²)(0.12 m)]½
= 1.534 m/sec The velocity just after the bullet is embedded in the block.
Before the bullet hits to just after the bullet is embedded in the block.
Use conservation of momentum:
⇒ (0.01 kg)v1i + 0 = (1.501 kg)(1.534 m/sec)
⇒ v1i = (1.501 kg)(1.534 m/sec)/(0.01 kg) = 230.2 m/sec
Before bullet is in the block to maximum height.
To get W use conservation of energy from before collision to maximum height.
½(0.01 kg)(230.2 m/sec)² + 0 + 0 + 0 = 0 + (1.51 kg)(9.8 m/sec²)(0.12 m) + W
⇒ W = 263.2 J
- A 300 g billiard ball going 0.5 m/sec collides head-on and elastically with a stationary ball that has three times the mass. What are the final velocities of each ball?
AnswerFrom conservation of energy when W = 0 (elastic collision), initial and final height is at zero, m2 = 3m1, v2i = 0:
½m1v1i² + 0 + 0 + 0 = ½m1v1f² + ½(3m1)v2f² + 0 + 0 + 0
v1i² = v1f² + 3v2f² ⇒ (0.5)² = v1f² + 3v2f²
From conservation of momentum:
m1v1i + 0 = m1v1f + 3m1v2f
v1i = v1f + 3v2f ⇒ 0.5 = v1f + 3v2f ⇒ v1f = 0.5 - 3v2f
Substitute v1f into the energy equation, and then solve for v2f:
⇒ (0.5)² = (0.5 - 3v2f)² + 3v2f² = (0.5)² - (2)(0.5)(3v2f) + (3v2f)² + 3v2f²
⇒ 0 = -3v2f + 12v2f² = (3)(-1 + 4v2f)(v2f)
The two roots of the equation are v2f = 0 (physically impossible), or v2f = 0.25.
v2f = 0.25 m/sec and v1f = 0.25 - 3v2f = 0.25 - 3(0.25) = -0.5 m/sec
Conservation of Energy with Rotational Kinetic Energy
- A 20 g disk with a radius of 1 cm is released from rest at the top of a smooth inclined plane, where the height from the bottom of the incline is 12 m. The disk reaches the bottom of the incline with a speed of 12 m/sec. What is the numerical value of the moment of inertia for this disk?
AnswerConservation of Energy ⇒ KEit + KEir + PEi = KEft + KEfr + PEf + W
⇒ ½mvi² + ½Iωi² + mghi = ½mvf² + ½Iωf² + mghf
Set h = 0 at the bottom of the incline. W = 0, vi = 0, ωi = 0, and hf = 0.
⇒ 0 + 0 + mghi = ½mvf² + ½Iωf² + 0
⇒ I = [mghi - ½mvf²]/[½ωf²] ; ωf = vf/r = (12 m/sec)/(0.01 m) = 1200 rad/sec
⇒ I = [(0.02 kg)(9.8 m/sec²)(12 m) - ½(0.02 kg)(12 m/sec)²]/[½(1200 rad/sec)²]
⇒ I = 1.27 x 10-6 kg-m²
- Numerical Answers
- 1.27 x 10-6 kg-m²
- What will the final speed be when a hollow sphere (I = ⅔mr²) is released from rest at a height of 80 cm and rolls down a 30 degree smooth incline?
EXTRA PROBLEMSAnswerConservation of Energy ⇒ KEit + KEir + PEi = KEft + KEfr + PEf + W
⇒ 0 + 0 + mghi = ½mvf² + ½Iωf² + 0 + 0
⇒ ghi = ½vf² + ½[⅔r²][vf/r]² = ½vf² + ⅓vf² = ⅚vf²
⇒ vf = [(6/5)ghi]½
⇒ vf = 3.07 m/sec
- Numerical Answers
- 3.07 m/sec
- A 300 g billiard ball going 0.10 m/sec collides head-on and elastically with a 350 g billiard ball going 0.13 m/sec in the opposite direction. What are the final velocities of each ball?
- What are the final momentums and velocities after a 0.22 kg air hockey puck initially traveling 0.4 m/sec at 45° collides with a 0.03 kg puck traveling at 0.3 m/sec at -30° (330° from the x-axis)?
- An airtrack car collides head-on and elastically with a second stationary car that has the same mass as the first car. Mathematically show that the final velocity of the first car is zero and that the final velocity of the second car is the same as the initial velocity of the first car.
AnswerFrom conservation of energy: W = 0 (elastic collision), initial and final height is along the track where we assign h equal to zero and v2i = 0:
½m1v1i² + 0 + 0 + 0 = ½m1v1f² + ½(m2)v2f² + 0 + 0 + 0
the one-half and the mass will cancel out, so
v1i² = v1f² + v2f²
From conservation of momentum:
m1v1i + 0 = m1v1f + m2v2f but m1 = m2, so
v1i = v1f + v2f ⇒ v1f = v1i - v2f and substituting into energy equation:
⇒ v1i² = (v1i - v2f)² + v2f² = v1i² - 2v1iv2f + v2f² + v2f²
⇒ 0 = 2v2f(v2f - v1i)
The two roots of the equation are v2f = 0 (physically impossible), or v2f = v1i.
⇒ v2f = v1i
AnswerFrom conservation of energy: W = 0 (elastic collision), initial and final height is along the track where we assign h equal to zero and v2i = 0:
½m1v1i² + 0 + 0 + 0 = ½m1v1f² + ½(m2)v2f² + 0 + 0 + 0
the one-half and the mass will cancel out, so
v1i² = v1f² + v2f²
From conservation of momentum:
m1v1i + 0 = m1v1f + m2v2f but m1 = m2, so
v1i = v1f + v2f ⇒ v2f = v1i - v1f and substituting into energy equation:
⇒ v1i² = v1f² + (v1i - v1f)² = v1f² + v1i² - 2v1iv1f + v1f²
⇒ 0 = 2v1f(v1f - v1i)
The two roots of the equation are v1f = v1i (physically impossible), or v1f = 0.
⇒ v1f = 0
Torque Problems
-
A 2 m long, 20 N uniform board is leaned against a smooth vertical wall, creating a 40° angle between the wall and the board. If the frictional force at the bottom of the board is 15 N, how high up the board, from the floor, could a 10 N box be placed without the board slipping?
Answer
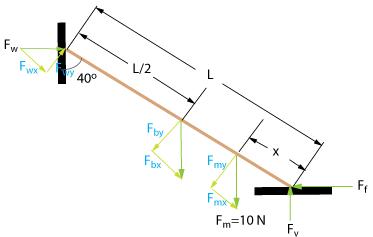
L = 2 m
Fb = 20 N
Fbx = (20 N)sin50° = 15.32 N
Fby = (20 N)cos50° = 12.86 NFmx = (10 N)sin50° = 7.66 N
Fmy = (10 N)cos50° = 6.43 N
Fwy = (15 N)sin50° = 11.49 NForces in x: Fw = Ff = 15 N
Forces in y: Fv = 20 N + 10 N = 30 N
Set l = 0 at the bottom. τin = τout ⇒ (Fwy)lw = (Fby)lb + (Fmy)lm
⇒ (11.49 N)(2 m) = (12.86 N)(1 m) + (6.43 N)(x) ⇒ x = 1.57 m
-
A straight, uniform ladder that is 2.5 m long is positioned against a wall. If both the wall has an upward frictional force of 15 N and the ground provides 20 N of horizontal frictional force and the ladder weighs 40 N, how high on the ladder can a 600 N person climb if the ladder makes a 35° angle with the wall?
Answer
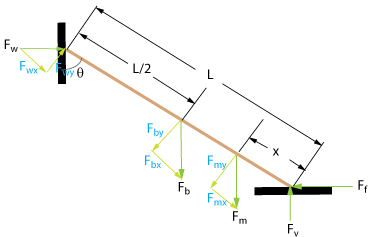
L = 2.5 m; θ=35°
Fb = 40 N
Fbx = (40 N)sin55°
= 32.77 N
Fby = (40 N)cos55°
= 22.94 NFm = 600 N
Fmx = (600 N)sin55° = 491.49 N
Fmy = (600 N)cos55° = 344.15 N
Fwy = (20 N)sin55° = 16.38 N
Fwf = 15 N (An upward frictional force at the wall, not shown in diagram.)
Fwfy = (15 N)cos35° = 12.28 NForces in x: Fw = Ff = 20 N
Forces in y: Fv + Fwfy = 40 N + 600 N = 640 NSet l = 0 at the bottom. τin = τout ⇒ (Fwy)lw + (Fwfy)lwf = (Fby)lb + (Fmy)lm
⇒ (16.38 N)(2.5 m) + (12.28 N)(2.5 m) = (22.94 N)(1.25 m) + (344.15 N)(x)
⇒ x = 0.125 m -
A 3m, uniform 50 N plank hangs 0.6 m over the side of a pirate ship. What is the maximum weight that a person can have without toppling the plank when standing on the end?
Answer
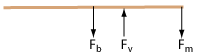
Fb = 50 N; Fm = ?
Forces in y: Fv = Fb + FmSet l = 0 at the Fv.
τin = τout ⇒ (Fm)lm = (Fb)lb ⇒ (Fm)(0.6 m) = (50 N)(0.9 m)
⇒ Fm = 75 N
-
Where is the center of mass of a nonuniform, 100 kg, 3 m long board that hangs 0.80 m over the edge of a platform while holding a maximum weight of 500 N on the extended end of the board?
Answer

Fb = (100 kg)(9.8 m/sec²) = 980 N; Fm = 500 N
Forces in y: Fv = Fb + FmSet l = 0 at the Fv.
τin = τout ⇒ (Fm)lm = (Fb)lb ⇒ (500 N)(0.8 m) = (980 N)(x)
⇒ x = 0.408 m⇒ xcm = 1.208 m from the end with the hanging mass.
-
What are the tensions, T1 and T2, in the supporting wires shown in Figure 7.2?
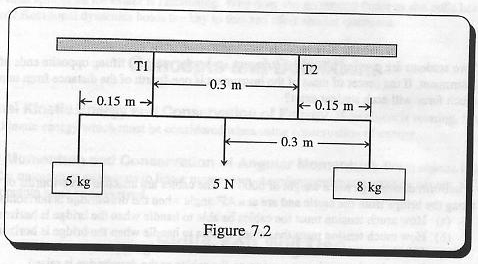 Answer
Answer5 kg ⇒ (5 kg)(9.8 m/sec²) = 49 N
8 kg ⇒ (8 kg)(9.8 m/sec²) = 78.4 N
Forces in y: T1 + T2 = 49 N + 5 N + 78.4 N = 132.4 NSet l = 0 at the T2.
τin = τout ⇒ (T1)(0.3 m) + (78.4 N)(0.15 m) = (5 N)(0.15 m) + (49 N)(0.45 m) ⇒ T1 = 36.8 N
⇒ T2 = 132.4 N - 36.8 N = 95.6 N
-
What is the tension in the horizontal wire connected to the wall in Figure 7.3 for a uniform supporting rod?
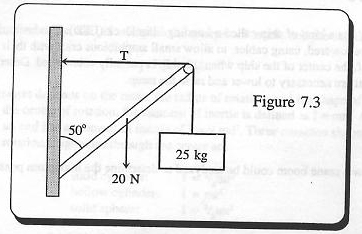 Answer
Answer25 kg ⇒ (25 kg)(9.8 m/sec²) = 245 N
Set the x-axis along the supporting rod. Tx = Tsin50° and Ty = Tcos50°
Set l = 0 at the bottom of the supporting rod.
τin = τout ⇒ [(20 N)cos40°](L/2) + [(245 N)cos40°](L) = (Ty)(L)
⇒ Ty = 195.3 N⇒ T = Ty/cos50° = 303.9 N
-
What is the tension shown in Figure 7.3 if the center of mass of the supporting rod is one-fourth of the way up from the wall, instead of at the center of the rod?
Answer
25 kg ⇒ (25 kg)(9.8 m/sec²) = 245 N
Set the x-axis along the supporting rod. Tx = Tsin50° and Ty = Tcos50°
Set l = 0 at the bottom of the supporting rod.
τin = τout ⇒ [(20 N)cos40°](L/4) + [(245 N)cos40°](L) = (Ty)(L)
⇒ Ty = 191.5 N⇒ T = Ty/cos50° = 297.9 N
EXTRA PROBLEMS
- Two children are sitting on either end of a seesaw. If one person weighs three-fourths as much as the other, where must they each sit in order to balance?
- Two students are moving a 500 N instrument across the room by lifting opposite ends of the instrument. If the center of mass of the instrument is one-fourth of the distance from one end, how much force will each student exert?
- A 30 kg uniform board that is 2 m long is placed on a horizontal table. Part of the length of the board overhangs the table. A 50 kg lamp is placed on the end of the board that extends out from the table. What is the maximum distance that the board can overhang and still stay on the table?
- A child's mobile is made by hanging a 2 g star and a 3 g moon on opposite ends of a 20 g uniform rod and hanging the rod with some string. If the rod is 30 cm long and hangs horizontally, where is the string attached?
- A 5 m rod has a 30 N mass hanging from one end. A fulcrum is placed 3 m from the end that has the 30 N mass. The center of mass of the rod is also 3 m from the 30 N mass. A second mass is placed at the opposite end of the rod from the 30 N mass. (a) What is the weight of the second mass? (b) If the rod is 50 N, what force does the fulcrum exert?
- A uniform drawbridge has a weight of 6000 N. The cables are attached three-fourths of the way along the bridge from the castle and are at a 45° angle when the drawbridge is horizontal. (a) How much tension must the cables be able to handle when the bridge is horizontal? (b) How much tension must the cables be able to handle when the bridge is horizontal and a 600 N person is standingat the end of the drawbridge opposite the castle? (c) Determine the maximum tension on the cables as the drawbridge is raised. (d) Show how the vertical force exerted by the castle on the bridge where they connect changes as the drawbridge is raised.
- Two billiard balls are traveling toward each other. A 500g billiard ball going 0.15 m/sec collides head-on and elastically with a 350 g billiard ball going 0.2 m/sec. What are the final velocities of each ball?